Below is a visual proof (!) that $32.5 = 31.5$. How could that be?
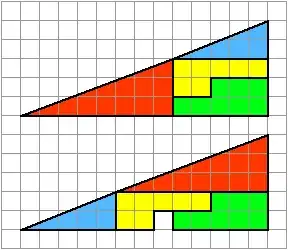
(As noted in a comment and answer, this is known as the "Missing Square" puzzle.)
Asked
Active
Viewed 1.1k times
73
Blue
- 69,412
- 11
- 108
- 217
Mehper C. Palavuzlar
- 2,026
- 4
- 23
- 21
-
2Everyone - further discussion on whether this is allowed can be found here: http://meta.math.stackexchange.com/questions/68/questions-about-math-jokes/70#70 – Edan Maor Jul 21 '10 at 08:16
-
3Compare the slope of the blue and red triangles. ${3\over 8} \neq {2\over 5}$ – Per Manne Apr 22 '13 at 14:43
-
See [here](https://web.archive.org/web/20100204093058/http://www.fantasticmetropolis.com/i/division/full/). – Andrés E. Caicedo Jul 20 '14 at 18:05
-
1To prove $31.5=32.5$, start from $88=89=90=91$ and subtract $56.5$, $57.5$, or $58.5$ http://math.harvard.edu/~elkies/Misc/rect2.pdf – Noam D. Elkies Feb 01 '15 at 22:18
8 Answers
98
Overlay the two triangles to see the difference. This is known as the missing square puzzle.
obataku
- 5,451
- 1
- 21
- 24
84
It's an optical illusion - neither the first nor second set of blocks actually describes a triangle. The diagonal edge of the first is slightly concave and that of the second is slightly convex.
To see clearly, look at the gradients of the hypotenuses of the red and blue triangles - they're not 'similar'.
gradient of blue triangle hypotenuse = 2/5
gradient of red triangle hypotenuse = 3/8
Since these gradients are different, combining them in the ways shown in the diagram does not produce an overall straight (diagonal) line.
Noldorin
- 6,184
- 6
- 32
- 37
-
20The word you call "gradient" is more familiar to me as "slope", but I guess it's just regional variation. – ShreevatsaR Aug 05 '10 at 16:25
-
6It's not exactly a coincidence that these are ratios of Fibonacci numbers. That's a good way to end up with ratios that are quite close to each other. In this case, both are approximants to $\frac{3-\sqrt{5}}{2}$, the limit of $\frac{F_n}{F_{n+2}}$. – 2'5 9'2 Feb 01 '15 at 08:42
55
Since this question has been bumped up, and some people find pictures helpful, may as well supply illustrations of the explanation.
The area of the red triangle is $\frac12(8)(3) = 12$ and that of the blue triangle is $\frac12(5)(2) = 5$. The area of the yellow and green regions are clearly 7 and 8 respectively, so the total coloured area is 12 + 5 + 7 + 8 = 32 which is less than 32.5, the area of a 13×5 triangle. Indeed, in the figure below you can see that the hypotenuses of the red and blue triangles "dip" below the actual hypotenuse of the large triangle; this accounts for the 0.5 difference in area.
Similarly, in the second figure, the hypotenuse of the red and blue triangles are above the actual hypotenuse, which accounts for the 0.5 difference in area again.
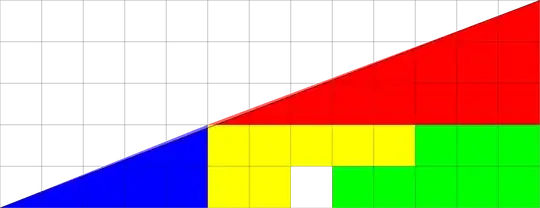
The difference between the two figures is a thin parallelogram with vertices at the endpoints of the hypotenuses in both figures (coloured pink below), which has area exactly 1. (If it looks much less, that's the nature of the optical illusion.)
Also, there are some links to similar figures at Wikipedia's article on this missing square puzzle.
ShreevatsaR
- 39,794
- 7
- 90
- 125
33
Surprisingly, the general case remains to be explained.
Consider the recursion $F(n+2) = F(n+1) + F(n)$, with $F(1) = F(2) = 1$
producing the Fibonacci numbers 1, 1, 2, 3, 5, 8, 13, 21, 34, 55, 89 ...
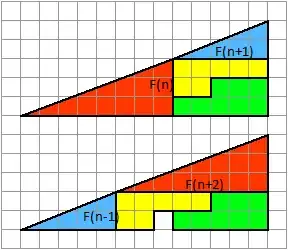
Let the blue triangle have horizontal and vertical sides of length $F(n+1)$ and $F(n-1)$, respectively.
Let the red triangle have horizontal and vertical sides of length $F(n+2)$ and $F(n)$, respectively.
So that
in the upper figure the two triangles create a rectangle with an area of $F(n)F(n+1)$
and
in the lower figure the two triangles create a rectangle with an area of $F(n-1)F(n+2)$
By the Fibonacci identity $F(n-1)F(n+2) - F(n)F(n+1) = \pm1$
we can see that the two rectangles are bound to differ in area by exactly one unit.
As n increases the gradient/slope of each triangle tends to the square of the Golden Ratio $ \phi$,
one from above and one from below.
Thus, as $\phi^2$ = 0.3819660112...
2/5 = 0.4 > $\phi^2$
3/8 = 0.375 < $\phi^2$
5/13 = 0.3846... > $\phi^2$
etc.
ThudanBlunder
- 569
- 4
- 9
-
As an aside, it's relatively harder to dissect the F(n)×F(n+1) and F(n-1)×F(n+2) rectangles into two shapes (which leave a "hole" in the second case), even though they differ in area by 1. (At least, I couldn't see something simple.) – ShreevatsaR Jun 04 '11 at 04:35
18
The red and blue triangles are not similar (the ratios of the sides are 3/8 = 0.375 and 2/5 = 0.4 respectively), so the "hypotenuse" of your big triangle is not a straight line.
Simon Nickerson
- 5,341
- 3
- 21
- 29
13
No, this is not a proof of your statement. If you look very closely, you will see that the hypotenuse of the triangles aren't straight. You can also verify this algebraically by calculating the internal angles of the triangle using trigonometry.
The other way to demonstrate the non-straightness of the hypotenuse is in the fact that the red and blue triangles aren't similar, which can be seen by the difference in the ratios of their width and height. For the blue triangle, this is 2/5 and for the red triangle this is 3/8. As they are both right angled triangles, these ratios would be the same for similar triangles and the fact that they aren't means the interior angles are different.
workmad3
- 1,508
- 11
- 14
1
I never looked at this, and thought that it was the same shape. You can't just change the number of angles in a shape and call it identical to the original. If you were to sketch only the outlines of each colored block on a blank piece of paper would the resulting "Shapes" be Identical or Even Similar? The answer is most definitely not. The illusion is looking at it on a graph VS in a Tangible form of any type, Imagine that you have 2 completely identical blocks of wood. You remove a identical portion as shown in figure 2, You didn't just make that hole appear out of nowhere because it can only be achieved by the removal of a block and thus a massive difference in the amounts of Angles laying within the "Shape" therefor making figure 2 nothing more than a combination of different shapes rather than being one definable shape of any type. I've yet to hear a better explanation than my own!
Mark Brauer
- 19
- 2
0
In the top diagram, the areas of the two smaller right triangles are (3)(8)/2 = 12, and (2)(5)/2 = 5 which total 17. The area of the rectangular region is (3)(5) = 15. The total area is therefore 17 + 15 = 32 [not 32.5]. In the bottom diagram, the same sum holds for the smaller triangles of 17, but now the rectangular region is (8)(2)=16, but wait! We have to subtract 1 because one of the little squares is missing. So the total area of the bottom diagram is 17 + 16 - 1 = 32. Therefore the areas of the two shapes are identical. The large "apparent triangle" of base 13 and height 5 and area 32.5 is not really a triangle at all because the two smaller triangles that form its hypotenuse have different slopes. It is a four sided figure.
