What is $dx$ in integration?

Question

When I was at school and learning integration in maths class at A Level my teacher wrote things like this on the board.

$$\int f(x)\, dx$$

When he came to explain the meaning of the $dx$, he told us "think of it as a full stop". For whatever reason I did not raise my hand and question him about it. But I have always shaken my head at such a poor explanation for putting a $dx$ at the end of integration equations such as these. To this day I do not know the purpose of the $dx$. Can someone explain this to me without resorting to grammatical metaphors?

Answer 1

The motivation behind integration is to find the area under a curve. You do this, schematically, by breaking up the interval $[a, b]$ into little regions of width $\Delta x$ and adding up the areas of the resulting rectangles. Here's an illustration from Wikipedia:

Then we want to make an identification along the lines of

$$\sum_x f(x)\Delta x\approx\int_a^b f(x)\,dx,$$

where we take those rectangle widths to be vanishingly small and refer to them as $dx$.

Answer 2

There are multiple ways of explaing what the $dx$ means.

• Practical explanation: It says we are integrating over variable $x$. If we were to integrate over variable $t$, we would write $dt$ instead, and so on.

• Infinitesimal explanation: We can think of an integral as the limit of a sum: The area under the graph of a (positive) function $f$ can be approximated by the sum $\sum_x f(x) \Delta x$, and in the limit, we make $\Delta x$ arbitrarily small and call it $dx$ (an "infinitesimal" quantity). Jonathan's answer explain that in detail.

• Advanced explanation: In vector analysis, $dx$ takes meaning as a differential form (roughly, something that behaves like an infinitesimaly small piece of a curve).

Answer 3

Leibniz, who introduced this notation in the 17th century, thought of $dx$ as an infinitely small increment of $x$, and at least as a heuristic, that is an immensely useful idea.

However, note some other points:

• $\displaystyle\int f(x,y)\,dx$ differs from $\displaystyle\int f(x,y)\,dy$. In one case, one integrates a function of $x$, and $y$ is constant; in the other these roles are reversed and one might be integrating a very different function.
• If $f(x)$ is in meters per second and $dx$ is in seconds, then $f(x)\,dx$ is in meters, and so is the integral. These things should be dimensionally correct, and are not so without the "$dx$".
• Sometimes one has a dot-product or a cross-product or a matrix product or some other sort of product between $f(x)$ and $dx$. How would one specify that without the "$dx$" written there?
• When doing substitutions, it becomes important to distinguish between $dx$ and $du$, etc.

Answer 4

Can someone explain this to me without resorting to grammatical metaphors?

It is a matter of grammar. The indefinite integral expression is a large expression organizing several pieces of information:

$$ \color{blue}\int \color{red}{\underline{\quad}} \color{green}d \color{purple}{\underline{\quad}} $$

The blue $\int$ is a symbol expressing that this is an integral expression. The rest of the expression is the integrand.

The integrand consists of three components: there is the green $d$ symbol. There is the purple slot on the right in which you place the name of the variable that you are integrating with respect to, and there is the red slot on the left in which you place the function expression you intend to integrate (with respect to the dummy variable).

There are other grammatical interpretations of integral expressions — most importantly (IMO) the notion of a "differential form" — but this is the one you are using in your introductory calculus class.

This particular grammatical form has some symbolism. It is a useful heuristic to think of a "$dx$" as a miniature variation in a function. You can extend this heuristic by imagining the integral to be "adding" up all of these miniature variations. The symbol $\int$, I believe, originated as an elongated $S$, for "sum"; not dissimilar to the choice of sigma ($\Sigma$) for summation expressions.

The notion of differential form is a very useful one you may be interested in learning more about. Unfortunately, I am not aware of any exposition that introduces it as applied to introductory calculus: it's usually only really introduced in a differential geometry course.

Answer 5

The $dx$ can be given various concrete meanings, none of which one can sensibly explain to someone first learning about integrals. It is, in reality, just a notation which comes to use from the originators of calculus, motivated by the ideas behind Jonathan's answer.

Today, the $dx$ serves the purpose of delimiting the integrand (although the physicists, rebellious as ever, like to write $\int\mathrm d xf(x)$ for what we write $\int f(x)\mathrm dx$...) and of making explicit the variable respect to which we are computing the integral (this s useful in situations like $\int f(x,y)\mathrm dx$, which is usually different from $\int f(x,y)\mathrm d y$)

As for concrete mathematical meanings: the $\mathrm dx$ can mean concretely all sort of things: the Lebesgue measure, a differential form, a density, and a few others. It would be impossible to explain what any of these mean to a student first encountering integrals!

Answer 6

Historically, calculus was framed in terms of infinitesimally small numbers. The Leibniz notation dy/dx was originally intended to mean, literally, the division of two infinitesimals. The Leibniz notation $\int f dx$ was meant to indicate a sum of infinitely many rectangles, each with infinitesimal width dx. (The integral sign $\int$ is an "S" for "sum.") Note that the factor $dx$ in the integral is needed in order to make the units come out right. For example, if you're calculating mechanical work as $W=\int F dx$, the units wouldn't be newton-meters if you didn't have the factor of $dx$, which has units of meters.

In the 19th century, mathematicians got uneasy about infinitesimals. They were afraid that a system of mathematics based on infinitesimals could not be developed in a fully rigorous and consistent way. Therefore, they rebuilt the foundations of calculus using limits, but they kept the Leibniz notation, which is extremely useful and practical. In this approach, $W=\int F dx$ stands for a limit of Riemann sums of rectangles with finite widths $\Delta x$, and the $dx$ becomes an archaism.

Around 1960, Abraham Robinson showed that it was possible to have calculus built on a foundation of infinitesimals, and that no inconsistency would result (unless there was an inconsistency that would also affect the real number system itself, which nobody thinks is the case). Therefore it's legitimate to think of integrals and derivatives in essentially the same way that Newton and Leibniz originally conceived of them -- in fact, scientists and engineers never actually stopped thinking about them that way.

Answer 7

Of course for something as simple as $\int{f(x)}dx$, you dont have to write $dx$ if you don't feel like it, and in many situations you are allowed to just write $\int{f}$, although I don't personally make a habit of it.

These things you ask about are not merely some convenient book-keeping device to let us know where the end of the intergral is, they are called differential forms, and you can add and multiply them together.

The algebra of differential forms follow naturally from the simple rule that $dx^2=0$ because this rule actually implies another very important rule, namely that $dx\wedge dy=-dy\wedge dx$, or in other words, that differential forms commute anti-symmetrically, see here for more info.

Answer 8

I once went at some length illustrating the point that for the purpose of evaluating integrals it is useful to look at $d$ as a linear operator.

Answer 9

I did the exact same question to myself and I give you the answer at which I arrived or how I see this.

Ok, let's see. The meaning of $dx$ on $\textit{definite}$ integrals is quite clear (as has been pointed out in other answers), it's the limit when the length element goes to $0$, so when writing $\int_0^1{}x^2dx$ the $dx$ has a clear meaning.

We know that $\textit{indefinite}$ integrals, or the anti-derivatives, can be used via calculus' fundamental theorem to calculate definite integrals, so one could think at this point that we write $dx$ while doing anti-derivatives because of the "closeness" of definite and indefinite integrals and that the $dx$ in $\int{}x^2dx$ does really have no other meaning than full stop.

But that's not the whole story. Truth is that the $dx$ is a "handy" way for changing variables (which is really useful doing integrals).

Imagine you wanna get the anti-derivative of $w(x)$. Since you wanna get the anti-derivative, this function is (you hope) a derivative of some (actually infinite but that is not important now) function, so you wanna integrate

$w(x)=f'(x)$

$f(x)$ IS what you wanna get.

Imagine also that you are incompetent enough not to know how to do this. So, in order to solve this you decide you wanna try changing variables, hoping that this will clear the mess and you will be more competent integrating respect to the new variable.

You proceed along this line defining the new variable

$x\equiv{}g(m)$ and $f(x)\equiv{}h(m)$

This is important, if we would be able to get $h(m)$ reversing the variable change we'd get $f(x)$ and the problem would be solved.

So you try out the new variable in hopes of getting $h'(m)$ from $f'(x)$ in hopes of being able to carry out the integration on $h'(m)$

$f'(x)=f'(x=g(m))=h'(m)m'(x)$

and remembering $m'(x)=\frac{dm}{dx}$ and rearranging terms

$f'(x)dx=h'(m)dm$

And now it is clear why the $dx$ is useful. Multiplying it with $f(x)$ makes the variables "ordered" after variable change and you easily get $h'(m)$ from $f'(x)$ which is what you wanted.

So you see, when doing variable change the $dx$ is something that helps you find the integrand respect to the new variable, and hence, it is written from the beginnig because it is expected that you will have to carry out variable changes and then you will need it.

So summarizing. It actually means full stop or absolutely nothing, but you will (probably) need it so write it and pretend not to see it until you need it.

Answer 10

It's very bad teaching to say that students should see the $dx $ part of an integral as something like a full-stop. Having met some poor math teachers myself (and some excellent ones, thankfully) I can empathize. Can I suggest that you just 'park' your old teacher's suggestion and look afresh - from a new perspective - at calculus ?

Before taking calculus, your should have been taught about the limits of functions. Otherwise differentiation and integration would be impossible to understand in the context of algebra and geometry. I strongly recommend Teach Yourself Calculus by P. Abbott. Old editions from 1960s are available on Amazon secondhand. (Please do NOT get the 'new' version by a chap called O'Neill - this is just a revenue hijack, as far as I can see.)

After getting a good handle on limits of functions, you'll soon see how $$ \lim_{\Delta x \to 0}{\frac{\Delta y}{\Delta x}} = \frac{dy}{dx}$$

In physical terms, $dx$ might be seen as the size of the biggest (but of course still quite miniscule in real-life terms) change to a variable, $x$, that will provide us with a means to make a precise estimation of the resulting change, $dy$, to a function $y = f(x)$. We can make this estimation using the differential of the function, $y = f(x)$, with respect to variable $x$.

$$ \frac{dy}{dx} = \frac{d[f(x)]}{dx} = f'(x) $$

Hence

$$ dy = (\frac{dy}{dx}) \ dx = f'(x) \ dx $$

Using algebra, we can work out what $ f'(x) $ or $ \frac{dy}{dx}$ is for any function via:

$$ f'(x) = \frac{dy}{dx} = \lim_{\Delta x \to 0}{\frac{f(x + \Delta x) - f(x)}{\Delta x}} $$

After doing a few of these one can set up a set of 'rules' by which different types of functions (e.g. polynomials, trigonometrics, product of functions, quotient of functions, etc) can have their differential function written out almost at sight. So if we are given a function $f(x)$ we can quickly write out its differential, $f'(x)$.

Differentials like $\frac{dy}{dx}$ tell us about the rate of change of a function compared to that of its dependent variable. But we sometimes need to do the opposite, i.e. find the function whose differential function, $f'(x)$, we happen to know. For example, we may need to find an overall change to that function as its dependent variable changes over a continuous range of values between $x_1$ and $x_2$. To do this we use the previous relation

$$ dy = d[f(x)] = f'(x) \ dx $$

This equation tells us that each part of the overall change in $y = f(x)$ as $x$ goes from $x_1$ to $x_2$ is the product of the rate of change of y with x evaluated at a point in that range multiplied by the differential in $x$, $dx$. Since we do not know the absolute value of $dx$ we cannot do it precisely by calculations of each $dy$. But we can use our 'rules' for differentiating a function in reverse to find the original function $f(x)$ whose $\frac{dy}{dx}$ equates to the function $f'(x)$. Applying $x_1$ and $x_2$ as arguments in this function will then provide us the overall change in $y$ as $x$ changes from $x_1$ to $x_2$, i.e.

$$ y = f(x) = \int{f'(x) \ dx}$$

where the $\int$ symbol signifies the reverse process (called integration) to the differentiation of a function.

Inserting $x_1$ and $x_2$ into the final function and subtracting gives the overall change in the function $y$ as $x$ changes from $x_1$ to $x_2$, i.e.

$$ \Delta y = f(x_2) - f(x_1) $$

So if your old teacher said that $dx$ in an integral should be seen as a full-stop, he was not correct. The $dx$ component must be there for the integral to have meaning since every integral is a product of a differential function and a differential in the dependent variable.

A thought has just occurred to me: perhaps your old teacher meant that there should be a full-stop between the $f(x)$ and the $dx$ ? That would make sense, as in algebra a dot (full-stop or period) is the operator for a multiplication - which is what $f(x) \ dx$ is when inside an integral . . .

$$ y = \int f'(x) \ . dx $$

I was going to suggest a thorough caning for that old teacher but now maybe the old devil wasn't so wrong after all . . . though this "insight" alone hardly takes us very far in understanding integrals.

Answer 11

An integral gives you the area between the horizontal axis and the curve. Most of the time this is the x axis.

                         y

                         |                    |
                       --|--              ----|---- f(x)
                     /   |   \          /     |
                    /    |     --------       |
          |        /     |                    |
     -----|-------       |                    |
          |              |                    |
          |              |                    |
----------|--------------+--------------------|----- x
        a                                   b

And the area enclosed is:

Area = $\int^b_a f(x) dx$

But say you didn't want to use an integral to measure the area between the x axis and the curve. Instead you just caclulate the average value of the graph between a and b and draw a striaght flat line y = avg(x) (the average value of x in that range).

Now you have a graph like this:

                         y

                         |                    |
                       - | -              - - | - - f(x)
          |          /   |   \          /     |
     -----|-----------------------------------|---- avg(x)
          |        /     |                    |
     - - -|- - - -       |                    |
          |              |                    |
          |              |                    |
----------|--------------+--------------------|----- x
        a                                   b

And the area enclosed is a rectangle:

Area = avg(x) w where w is the width iof the section

The height is avg(x) and the width is w = b-a or in English, "the width of a slice of the x axis going from a to b."

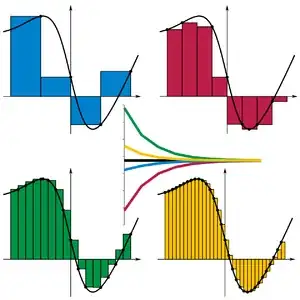
But say you need a more accurate area. You could break the graph up into smaller sections and make rectangles out of them. Say you make 4 equal sections:

                           y

                           |                    |
                      |----|---|        |-------|---- f(x)
                      |    |   |        |       |
                      |    |   |--------|       |
            |         |    |   |        |       |
       -----|---------|    |   |        |       |
            |         |    |   |        |       |
            |         |    |   |        |       |
  ----------|---------|----+---|--------|-------|----- x
            a                                   b

And the area is:

Area = section 1 + section 2 + section 3 + section 4

= avg(x,1) w + avg(x,2) w + avg(x,3) w + avg(x,4) w

where w is the width of each section. The sections are all the same size, so in this case w=(b-a)/4 or in English, "the width of a thin slice of the x axis or 1/4 of the width from a to b."

And if we write this with a sumation we get:

Area = $\sum^4_{n=1}avg(x,n) w$

But it's still not accurate enough. Let's use an infinite number of sections. Now our area becomes a summation of an infinite number of sections. Since it's an infinite sum, we will use the integral sign instead of the summation sign:

Area = $\int avg(x) w$

where avg(x) for an infinitely thin section will be equal to f(x) in that section, and w will be "the width of an infinitely thin section of the x axis."

So instead of avg(x) we can write f(x), because they are the same if the average is taken over an infinitely small width.

And we can rename the w variable to anything we want. The width of a section is the difference between the right side and the left side. The difference between two points is often called the delta of those values. So the difference of two x values (like a and b) would be called delta-x. But that is too long to use in an equation, so when we have an infinitely small delta, it is shortened to dx.

If we replace avg(x) and w with these equivalent things:

Area = $\int f(x) dx$

So what the equation says is:

Area equals the sum of an infinite number of rectangles that are f(x) high and dx wide (where dx is an infinitely small distance).

So you need the dx because otherwise you aren't summing up rectangles and your answer wouldn't be total area.

dx literally means "an infinitely small width of x".

It even means this in derivatives. A derivative of a function is the slope of the graph at that point. Slope is usually measured as the y difference of two points divided by the x difference of those points:

Slope = (y2 - y1) / (x2 - x1)

But the closer these points get the smaller these differences get. Let's start calling them deltas, because the difference between two points is often called the delta of those values.

Slope = delta-y / delta-x

The deltas get smaller and smaller as these two x,y points get closer and closer. When they are an infinitely small distance apart, then the delta-y and delta-x is shortened to dy and dx:

Slope = dy / dx

The slope is still Slope = (y2 - y1) / (x2 - x1) but these points are infinitely close together, so we use dy and dx to tell ourselves that they are infinitely close or "differential distances."

Answer 12

The reason why dx is added to after the integrand is as follows:

Say, that dy/dx = f(x). Then, dy= f(x) * dx. So, y= int (f(x)*dx)

Therefore the dx has to be part of the expression if y is to be calculated.